Mastering Mental Math: How to Multiply Any Number by 11 Effortlessly
Written on
Chapter 1: Introduction to Mental Multiplication
Are you in search of a quick and easy way to multiply any number by 11 in your mind? If so, you’ve come to the right spot! Beyond a joke, any rapid calculation technique that can be executed mentally often proves to be highly effective as a computational algorithm for specific tasks. Therefore, it’s likely more beneficial than most people realize. In this article, I will showcase a swift calculation method involving the number 11, relying solely on addition. Yes, you heard it right—addition is all you need! Let’s see just how simple this method can be.
Section 1.1: Multiplying Two-Digit Numbers by 11
Let's begin with how to multiply 2-digit numbers by 11. For example, if we calculate 11 * 81, the result is 891. While this might not be straightforward for everyone using traditional multiplication techniques, we can simplify the process.
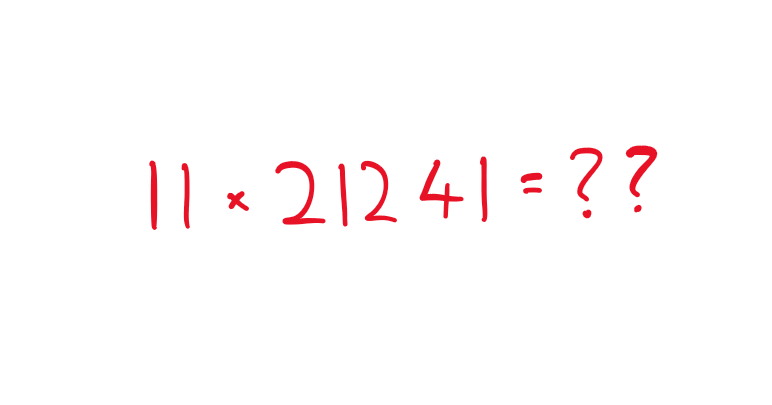
In fact, we can break it down algebraically, but that can still be somewhat cumbersome. The technique I’m about to share is much faster and relies only on addition. Let’s illustrate this method step-by-step.
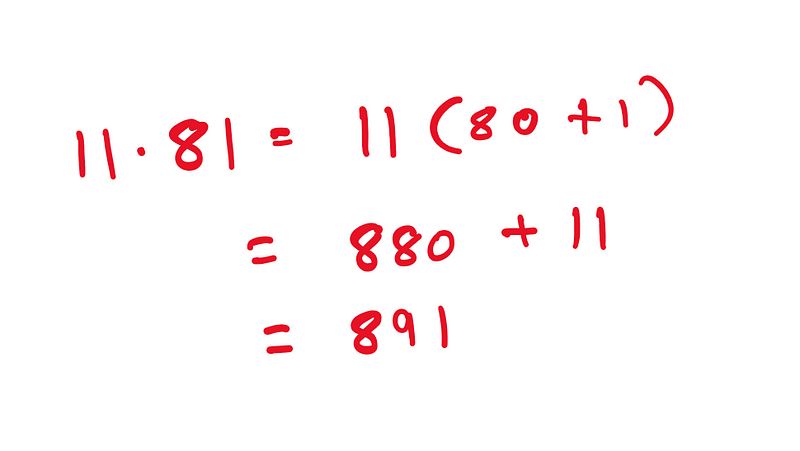
To summarize the steps:
- Write the two digits of the number separately with a space in the middle.
- Add the two digits together and place the sum in between.
And just like that, you have your multiplication result! While it seems straightforward, there are some nuances to address, especially when multiplying 3-digit numbers.
Section 1.2: Multiplying Three-Digit Numbers by 11
Now let's explore multiplying 3-digit numbers by 11. The same fundamental approach applies, with a slight modification.
Consider the example of 11 * 217. Using standard methods, this multiplication could be tricky. Let’s apply our new technique here:
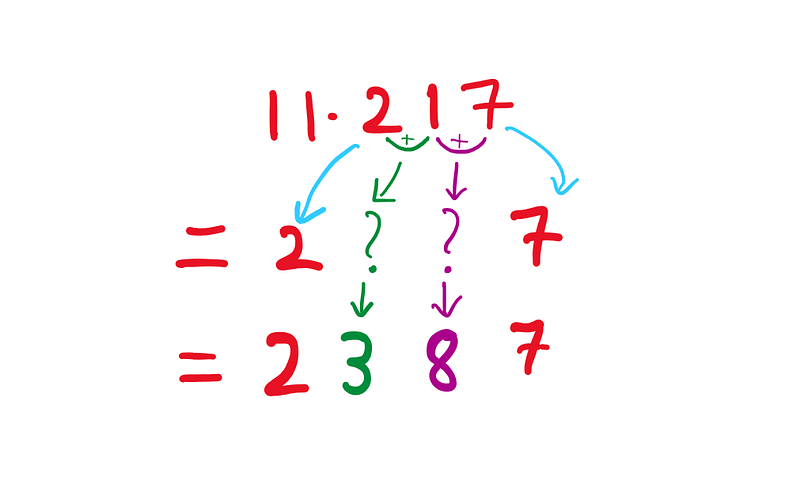
Here’s how to approach it in three steps:
- Write the first and last digits of the number separately, leaving enough space for two digits in the middle.
- Add the first digit to the middle digit and write the result as the second digit.
- Finally, add the middle digit to the last digit and record that as the third digit.
This method simplifies the process, but it raises a question: how do we scale this technique for larger numbers? Let’s tackle that next.
Chapter 2: Expanding to Any Number
Let’s take the example of multiplying 11 * 21241. As before, I will first demonstrate the method and then clarify the underlying principles:
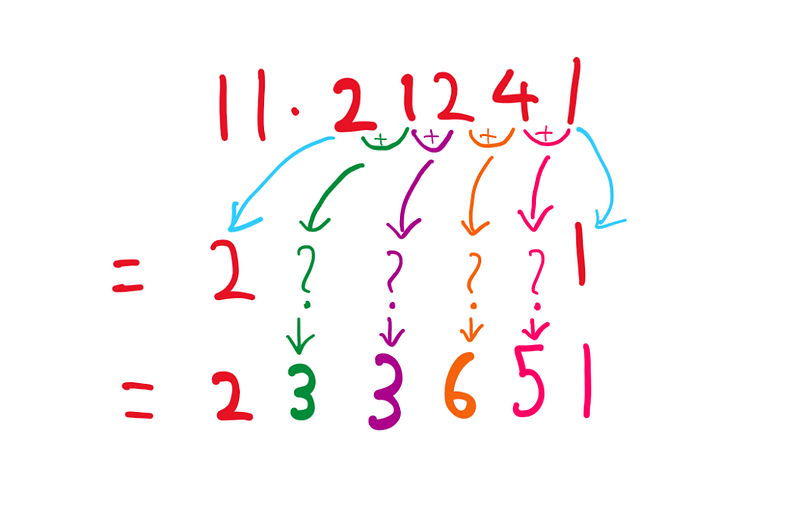
In this case:
- We write the first and last digits of the original number as the first and last digits of the result, leaving a gap in between.
- The gap will accommodate the number of addition pairs from left to right. For 21241, we have four pairs: 2+1, 1+2, 2+4, and 4+1.
Before you start celebrating your newfound skill, there’s one last detail to consider.
Section 2.1: Understanding Carry Over
When adding two digits, everything works smoothly as long as the sum is a single digit (e.g., 2+4 = 6). However, if you encounter a sum that results in a two-digit number (like 7+4 = 11), you must carry over the first digit to the left.
Let’s work through a couple of examples to illustrate this:
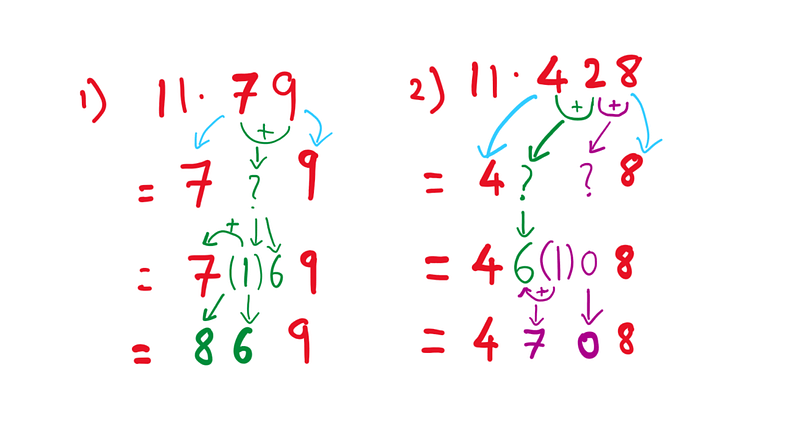
And there you have it! You can now confidently multiply any number by 11 in your head.
I hope you found this guide both engaging and informative. If you want to support my work further, consider clapping, following, and subscribing to my content.
For additional reading, you might enjoy articles like "Why Is 3 A Special Denominator in Division?" and "How Many Decimal Digits of Pi Do We Really Need?"
This video, titled "90% Don't Know This Math Secret - How to Multiply by 11," explores this mental math trick in detail, making it easier to grasp.
In this short clip, "Cool Mental Math Trick for 11's | Multiplying 2-Digit Numbers by 11 | Math with Mr. J," you can see the method demonstrated in action for quick understanding.